Thư Viện Bài Giảng Vật Lý Đại Cương
1.1. Current Electricity
A. Introduction
An electrical circuit consists of some active and passive elements. The active elements such as a battery or a cell, supply electric energy to the circuit. On the contrary, passive elements consume or store the electric energy. The basic passive elements are resistor, capacitor and inductor.
A resistor opposes the flow of current through it and if some current is passed by maintaining a potential difference across it, some energy is dissipated in the form of heat. A capacitor is a device which stores energy in the form of electric potential energy. It opposes the variations in voltage. An inductor opposes the variations in current. It does not oppose the steady current through it.
Fundamentally, electric circuits are a means for conveying energy from one place to another. As charged particles move within a circuit, electric potential energy is transferred from a source (such as a battery or a cell) to a device in which that energy is either stored or converted to another form, like sound in a stereo system or heat and light in a toaster or light bulb. Electric circuits are useful because they allow energy to be transported without any moving parts (other than the moving charged
particles themselves).
In this chapter, we will study the basic properties of electric currents. We’ll study the properties of batteries and how they cause current and energy transfer in a circuit. In this analysis, we will use the concepts of current, potential difference, resistance and electromotive force.
Electric Current
Flow of charge is called electric current. The direction of electric current is in the direction of flow of positive charge or in the opposite direction of flow of negative charge.
Current is defined quantitatively in terms of the rate at which net charge passes through a cross-section area of the conductor.
Thus, \( I=\frac{d q}{d t} \quad \text { or } \quad i=\frac{d q}{d t} \)
We can have the following two concepts of current, as in the case of velocity, instantaneous current and average current.
Instantaneous current \( =\frac{d q}{d t}= \) current at any point of time and
Average current \( =\frac{q}{t} \)
Hence-forth unless otherwise referred to, current would signify instantaneous current. By convention, the direction of the current is assumed to be that in which positive charge moves. In the SI system, the unit of current is ampere (A).
\( 1 \mathrm{~A}=1 \mathrm{C} / \mathrm{s} \)
Household currents are of the order of few amperes.
Nhận Dạy Kèm Vật Lý Đại Cương Online qua ứng dụng Zoom, Google Meet,...
- Dạy kèm tương tác 1 thầy 1 trò! Hỗ trợ trực tuyến 24/7
- Dạy kèm Vật Lý Đại Cương (Cơ - Nhiệt - Điện Từ - Quang - VLNT-HN)
- Bồi dưỡng HSG Vật lý các cấp, luyện thi vào lớp 10 Chuyên, ôn thi ĐH-CĐ
- Sách Giải Bài Tập Vật Lý Đại Cương - Vật Lý Kỹ Thuật - Vật Lý Lý Thuyết
- Lịch học sắp xếp linh động, sáng - chiều - tối đều học được!
- Thời gian học từ 1,5h - 2h/1 buổi!
Flow of Charge
If current is passing through a wire then it implies that a charge is flowing through that wire. Further,
\( i=\frac{d q}{d t} \Rightarrow d q=i d t \) (i)
Now, three cases are possible :
Case 1: If given current is constant, then from Eq. (i) we can see that flow of charge can be obtained directly by multiplying that constant current with the given time interval. Or,
\( \Delta q=i \times \Delta t \)
Case 2: If given current is a function of time, then charge flow can be obtained by integration. Or,
\( \Delta q=\int_{t_i}^{t_f} i d t \)
Case 3: If current versus time is given, then flow of charge can be obtained by the area under the graph.
\( \Delta q=\text { area under } i-t \text { graph } \)
Extra Points to Remember
- The current is the same for all cross-sections of a conductor of non-uniform cross-section. Similar to the water flow, charge flows faster where the conductor is smaller in cross-section and slower where the conductor is larger in cross-section, so that charge rate remains unchanged.
- Electric current is very similar to water current, consider a water tank kept at some height and a pipe is connected to the water tank. The rate of flow of water through the pipe depends on the height of the tank. As the level of water in the tank falls, the rate of flow of water through the pipe also gets reduced. Just as the flow of water depends on the height of the tank or the level of water in the tank, the flow of current through a wire depends on the potential difference between the end points of the wire. As the potential difference is changed, the current will change. For example, during the discharging of a capacitor potential difference and hence, the current in the circuit decreases with time. To maintain a constant current in a circuit a constant potential difference will have to be maintained and for this a battery is used which maintains a constant potential difference in a circuit.
Though conventionally a direction is associated with current (opposite to the motion of electrons), it is not a vector as the direction merely represents the sense of charge flow and not a true direction. Further, current does not obey the law of parallelogram of vectors, i.e. if two currents \( i_1 \) and \( i_2 \) reach a point we always have \( i=i_1+i_2 \) whatever be the angle between \( i_1 \) and \( i_2 \).
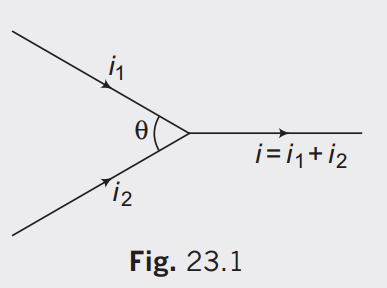
According to its magnitude and direction, current is usually divided into two types:
(i) Direct current (DC) If the magnitude and direction of current does not vary with time, it is said to be direct current (DC). Cell, battery or DC dynamo are its sources.
(ii) Alternating current (AC) If a current is periodic (with constant amplitude) and has half cycle positive and half negative, it is said to be alternating current (AC). AC dynamo is the source of it.
- If a charge \( q \) revolves in a circle with frequency \( f \), the equivalent current, \( i=q f\)
- In a conductor, normally current flow or charge flow is due to flow of free electrons.
- Charge is quantised. The quantum of charge ise. The charge on any body will be some integral multiple of e, i.e. \( q= \pm n e \)
where, \( n=1,2,3 \ldots \)
B. Example
Example 1. In a given time of \( 10 \mathrm{~s}, 40 \) electrons pass from right to left. In the same interval of time 40 protons also pass from left to right. Is the average current zero? If not, then find the value of average current.
Solution:
No, the average current is not zero. Direction of current is the direction of motion of positive charge or in the opposite direction of motion of negative charge. So, both currents are from left to right and both currents will be added.
\( \begin{aligned} I_{\mathrm{av}} & =I_{\text {electron }}+I_{\text {proton }} \\ & =\frac{q_1}{t_1}+\frac{q_2}{t_2} \\ & =\frac{40 e}{10}+\frac{40 e}{10} \\ & =8 e \\ & =8 \times 1.6 \times 10^{-19} \mathrm{~A} \\ & =1.28 \times 10^{-18} \mathrm{~A} \end{aligned} \quad \)
Example 2. A constant current of 4 A passes through a wire for \( 8 \mathrm{~s} \). Find total charge flowing through that wire in the given time interval.
Solution:
Since, \( i= constant \)
\( \begin{aligned} \therefore \quad \Delta q & =i \times \Delta t \\ & =4 \times 8 \\ & =32 \mathrm{C} \end{aligned} \)
Sách University Physics - Electricity and Magnetism
Example 3.A wire carries a current of 2.0 A. What is the charge that has flowed through its cross-section in \( 1.0 s \) ? How many electrons does this correspond to?
Solution:
\( \because \quad i=\frac{q}{t} \)
\( \begin{aligned}\therefore \quad q & =i t=(2.0 \mathrm{~A})(1.0 \mathrm{~s})=2.0 \mathrm{C} \\ \therefore \quad q & =n e \\ n & =\frac{q}{e}=\frac{2.0}{1.6 \times 10^{-19}} \\ & =1.25 \times 10^{19} \end{aligned} \)
Example 4. The current in a wire varies with time according to the relation
\( i=(3.0 \mathrm{~A})+(2.0 \mathrm{~A} / \mathrm{s}) t \)
(a) How many coulombs of charge pass a cross-section of the wire in the time interval between \( t=0 \) and \( t=4.0 \mathrm{~s} \) ?
(b) What constant current would transport the same charge in the same time interval?
Solution:
(a)
\( \begin{aligned}& i=\frac{d q}{d t} \\& \int_0^q d q=\int_0^4 i d t \\& \therefore \quad q=\int_0^4(3+2 t) d t \\& =\left[3 t+t^2\right]_0^4=[12+16] \\& =28 \mathrm{C} \\&\end{aligned} \)
(b) \( i=\frac{q}{t}=\frac{28}{4}=7 \mathrm{~A} \)
Example 5. Current passing through a wire decreases linearly from \( 10 \mathrm{~A} \) to 0 in 4 s. Find total charge flowing through the wire in the given time interval.
Solution:
Current versus time graph is as shown in figure.
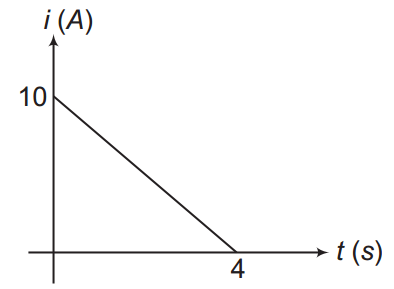
Area under this graph will give us net charge flow.
Hence,
\( \begin{aligned}\Delta q & =\text { Area } \\& =\frac{1}{2} \times \text { base } \times \text { height } \\ & =\frac{1}{2} \times 4 \times 10 \\& =20 \mathrm{C}\end{aligned} \)
C. INTRODUCTORY EXERCISE
1. How many electrons per second pass through a section of wire carrying a current of \( 0.7 \mathrm{~A} \) ?
2. A current of 3.6 A flows through an automobile headlight. How many coulombs of charge flow through the headlight in \( 3.0 \mathrm{~h} \) ?
3. A current of \( 7.5 \mathrm{~A} \) is maintained in wire for \( 45 \mathrm{~s} \). In this time,
(a) how much charge and
(b) how many electrons flow through the wire?
4. In the Bohr model, the electron of a hydrogen atom moves in a circular orbit of radius \( 5.3 \times 10^{-11} \mathrm{~m} \) with a speed of \( 2.2 \times 10^6 \mathrm{~m} / \mathrm{s} \). Determine its frequency \( f \) and the current \( I \) in the orbit.
5. The current through a wire depends on time as, \( i=(10+4 t) \)
Here, \( i \) is in ampere and \( t \) in seconds. Find the charge crossed through a section in time interval between \( t=0 \) to \( t=10 \mathrm{~s} \).
6. In an electrolyte, the positive ions move from left to right and the negative ions from right to left. Is there a net current? If yes, in what direction?
Trong quá trình đăng tải bài viết lên website không thể tránh khỏi việc sai sót, nên bạn đọc muốn xem đầy đủ các dạng bài tập giải chi tiết hãy vui lòng mua sách University Physics – Electricity and Magnetism!
Các bài viết cùng chủ đề!
Các Sách Giải Bài Tập - Đề Thi do Trung tâm phát hành!
Thư Viện Bài Giảng Vật Lý Đại Cương được xây dựng trên WordPress










